views
- Find the constant of proportionality using the equation when ratios are directly proportional (both increase at the same rate).
- Find the constant of proportionality using the equation when ratios are inversely proportional (one increases as the other decreases at the same rate).
- Use the constant of proportionality to determine what value will be at any value of so you can graph the coordinates.
What is the constant of proportionality?
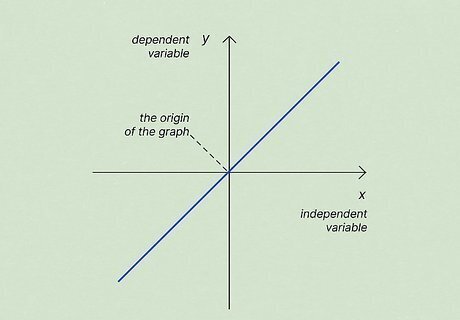
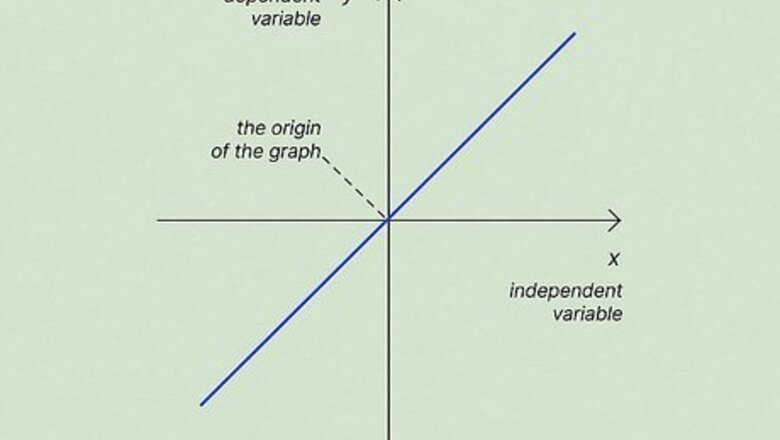
The constant of proportionality is the rate at which the x and y variables change. In other words, this is a constant slope. When two variables are proportional, that means they both change at the same rate. If you plot them on a graph, you can draw a straight line between all of the points that goes through the origin of the graph. The constant of proportionality is the slope of that line. Graphed coordinates ( x , y ) {\displaystyle (x,y)} (x,y) can also be written as a ratio y : x {\displaystyle y:x} {\displaystyle y:x} or a fraction y x {\displaystyle {\frac {y}{x}}} {\displaystyle {\frac {y}{x}}}. You can also think of x {\displaystyle x} x as the independent variable and y {\displaystyle y} y as the dependent variable, since y {\displaystyle y} y changes in relation to the change in x {\displaystyle x} x. The constant of proportionality can never be 0 {\displaystyle 0} {\displaystyle 0}.
Types of Proportionality
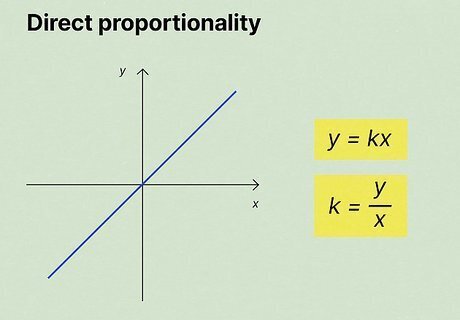
Direct proportionality: If two variables both increase at the same rate, they have a direct proportional relationship. Direct proportionality is represented by the equation y = k x {\displaystyle y=kx} y=kx where k {\displaystyle k} k is the constant of proportionality. The same equation can also be written k = y x {\displaystyle k={\frac {y}{x}}} {\displaystyle k={\frac {y}{x}}}. Use this version if you're trying to find the constant of proportionality and already have the values for the two variables. The formula d = r t {\displaystyle d=rt} {\displaystyle d=rt} (distance = rate x time), which you might recognize from science class, is another version of the equation for the constant of proportionality.
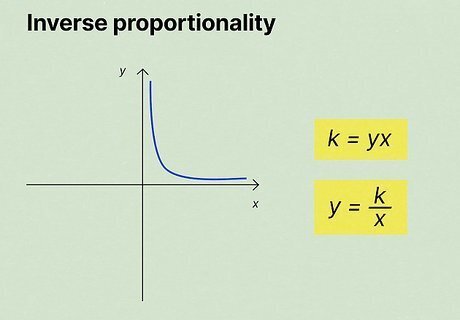
Inverse proportionality: Ratios are inversely proportional if one amount decreases at the same rate that the other increases. Inverse proportionality is represented by the equation y = k x {\displaystyle y={\frac {k}{x}}} {\displaystyle y={\frac {k}{x}}} where k {\displaystyle k} k is the constant of proportionality. The same equation can also be written k = y x {\displaystyle k=yx} {\displaystyle k=yx}. Use this version to find the constant of proportionality when you already have the values for the other two variables.
Finding the Constant of Proportionality with Ratios
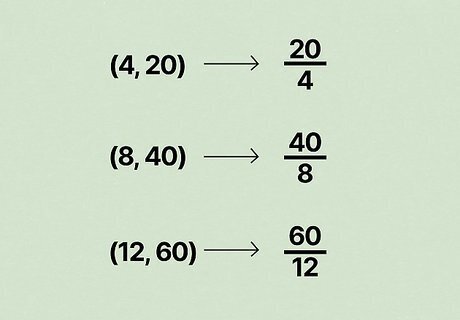
Set up each ratio as a fraction. In a ratio, the first number is the denominator of the fraction and the second number is the numerator. On a graph, the x {\displaystyle x} x coordinate of any point is the denominator, while the y {\displaystyle y} y coordinate is the numerator. For example, the ordered pairs ( 4 , 20 ) {\displaystyle (4,20)} {\displaystyle (4,20)}, ( 8 , 40 ) {\displaystyle (8,40)} {\displaystyle (8,40)}, and ( 12 , 60 ) {\displaystyle (12,60)} {\displaystyle (12,60)} are the same as the ratios 20 : 4 {\displaystyle 20:4} {\displaystyle 20:4}, 40 : 8 {\displaystyle 40:8} {\displaystyle 40:8}, and 60 : 12 {\displaystyle 60:12} {\displaystyle 60:12}. As fractions, they are 20 4 {\displaystyle {\frac {20}{4}}} {\displaystyle {\frac {20}{4}}}, 40 8 {\displaystyle {\frac {40}{8}}} {\displaystyle {\frac {40}{8}}}, and 60 12 {\displaystyle {\frac {60}{12}}} {\displaystyle {\frac {60}{12}}}.
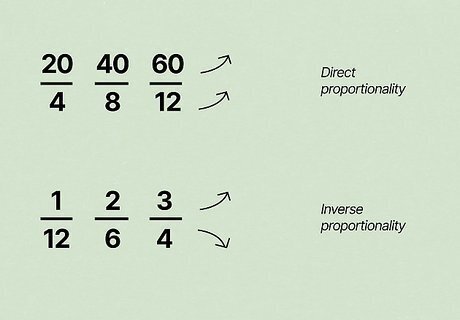
Determine whether you're looking at direct or inverse proportionality. Look at the numbers in the set of ratios you've been given. If they all increase, they're directly proportional. On the other hand, if one set of values increases and the other set of values decreases, you've got inverse proportionality. For example, look at the set 20 4 {\displaystyle {\frac {20}{4}}} {\displaystyle {\frac {20}{4}}}, 40 8 {\displaystyle {\frac {40}{8}}} {\displaystyle {\frac {40}{8}}}, and 60 12 {\displaystyle {\frac {60}{12}}} {\displaystyle {\frac {60}{12}}}. In each ratio, the values of the numerator and the denominator both increase, so you're looking at direct proportionality. What if you had 1 12 {\displaystyle {\frac {1}{12}}} {\frac {1}{12}}, 2 6 {\displaystyle {\frac {2}{6}}} {\frac {2}{6}}, and 3 4 {\displaystyle {\frac {3}{4}}} {\displaystyle \frac{3}{4}}? The values of the denominator decrease as the values of the numerator increase, so you're looking at inverse proportionality.
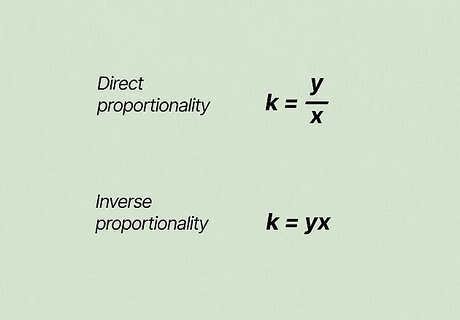
Plug the values from one of the ratios into the proper equation. If the ratios have direct proportionality, use the equation k = y x {\displaystyle k={\frac {y}{x}}} {\displaystyle k={\frac {y}{x}}}. For inverse proportionality, use the equation k = y x {\displaystyle k=yx} {\displaystyle k=yx}. To continue from the previous example, take the first ratio of 20 4 {\displaystyle {\frac {20}{4}}} {\displaystyle {\frac {20}{4}}}. Since the set is directly proportional, you'd use k = 20 4 = 5 {\displaystyle k={\frac {20}{4}}=5} {\displaystyle k={\frac {20}{4}}=5}. Your constant of proportionality is 5 {\displaystyle 5} 5. What about the inverse set? Take the ratio 1 12 {\displaystyle {\frac {1}{12}}} {\frac {1}{12}} and plug the numbers into the equation k = y x {\displaystyle k=yx} {\displaystyle k=yx} to get k = ( 1 ) ( 12 ) = 12 {\displaystyle k={(1)}{(12)}=12} {\displaystyle k={(1)}{(12)}=12}. Your constant of proportionality is 12 {\displaystyle 12} 12.
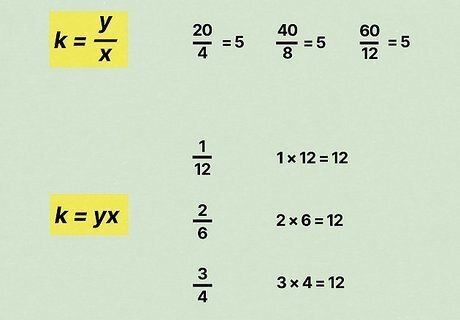
Check your work with the other ratios. If the ratios are proportional, the constant of proportionality will be the same for all of them. If any of them are different, then the ratios aren't proportional and there is no constant of proportionality. Try this on your own with the ratios in the set 20 4 {\displaystyle {\frac {20}{4}}} {\displaystyle {\frac {20}{4}}}, 40 8 {\displaystyle {\frac {40}{8}}} {\displaystyle {\frac {40}{8}}}, and 60 12 {\displaystyle {\frac {60}{12}}} {\displaystyle {\frac {60}{12}}}. You'll see that you get 5 {\displaystyle 5} 5 for each ratio, which tells you this ratios are indeed directly proportional! This also applies to ratios that are inversely proportional. For example, in the set 1 12 {\displaystyle {\frac {1}{12}}} {\frac {1}{12}}, 2 6 {\displaystyle {\frac {2}{6}}} {\frac {2}{6}}, and 3 4 {\displaystyle {\frac {3}{4}}} {\displaystyle \frac{3}{4}}, plugging each ratio into the equation k = y x {\displaystyle k=yx} {\displaystyle k=yx} gets you a constant of 12 {\displaystyle 12} 12 for each ratio, so they are inversely proportional.
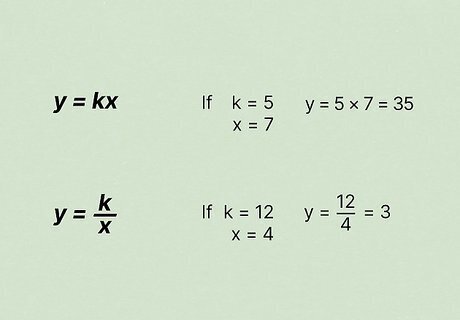
Determine other values in the series using the constant of proportionality. Now that you know the constant of proportionality, you can figure out what the value of y {\displaystyle y} y would be for any value of x {\displaystyle x} x. Use the equation y = k x {\displaystyle y=kx} y=kx if the set of ratios is directly proportional. If x {\displaystyle x} x is 7 {\displaystyle 7} 7 and k {\displaystyle k} k is 5 {\displaystyle 5} 5, y {\displaystyle y} y would be 35 {\displaystyle 35} 35 ( y = ( 7 ) ( 5 ) = 35 {\displaystyle y=(7)(5)=35} {\displaystyle y=(7)(5)=35}). Use the equation y = k x {\displaystyle y={\frac {k}{x}}} {\displaystyle y={\frac {k}{x}}} if the set of ratios is inversely proportional. If x {\displaystyle x} x is 4 {\displaystyle 4} 4 and the constant of proportionality is 12 {\displaystyle 12} 12, y {\displaystyle y} y would be 3 {\displaystyle 3} 3 ( y = 12 4 = 3 {\displaystyle y={\frac {12}{4}}=3} {\displaystyle y={\frac {12}{4}}=3}).
Practice Problems

Find the constant of proportionality for 10.5 1.5 {\displaystyle {\frac {10.5}{1.5}}} {\displaystyle {\frac {10.5}{1.5}}}, 14 2 {\displaystyle {\frac {14}{2}}} {\displaystyle {\frac {14}{2}}}, 24.5 3.5 {\displaystyle {\frac {24.5}{3.5}}} {\displaystyle {\frac {24.5}{3.5}}}, and 35 5 {\displaystyle {\frac {35}{5}}} {\frac {35}{5}}. Hint: All of the values increase, so use the equation for direct proportionality: k = y x {\displaystyle k={\frac {y}{x}}} {\displaystyle k={\frac {y}{x}}}.

Find the constant of proportionality for 105 − 2 {\displaystyle {\frac {105}{-2}}} {\displaystyle {\frac {105}{-2}}}, − 105 2 {\displaystyle {\frac {-105}{2}}} {\displaystyle {\frac {-105}{2}}}, − 35 6 {\displaystyle {\frac {-35}{6}}} {\displaystyle {\frac {-35}{6}}}, − 21 10 {\displaystyle {\frac {-21}{10}}} {\displaystyle {\frac {-21}{10}}}, and − 15 14 {\displaystyle {\frac {-15}{14}}} {\displaystyle {\frac {-15}{14}}}. Hint: The y {\displaystyle y} y values are decreasing, so use the equation for inverse proportionality: k = y x {\displaystyle k=yx} {\displaystyle k=yx}.

The slowest mammal in the world, the sloth, moves at a rate of 6 {\displaystyle 6} 6 feet per minute. How far will Flash the sloth have gone in 5 {\displaystyle 5} 5 minutes? And how long will it take Flash to complete the 100-yard dash? Hint: 100 yards is 300 feet.

You're on a road trip. Each time you get gas, you record the number of miles you drove and the amount of gas your car used: 224 {\displaystyle 224} {\displaystyle 224} miles on 8 {\displaystyle 8} 8 gallons, 280 {\displaystyle 280} {\displaystyle 280} miles on 10 {\displaystyle 10} 10 gallons, and 112 {\displaystyle 112} {\displaystyle 112} miles on 4 {\displaystyle 4} 4 gallons. What is your car's gas mileage? Hint: Gas mileage is the number of miles your car can drive on one gallon of gas, so you're looking for the constant of proportionality here.
Solutions to Practice Problems
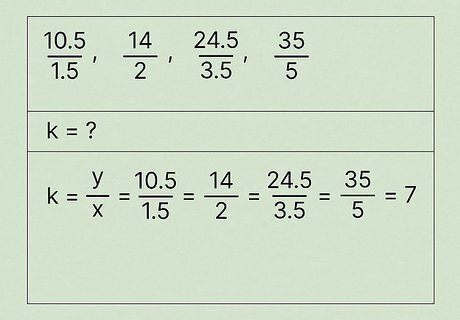
The constant of proportionality is 7 {\displaystyle 7} 7. If you remember your multiplication tables, you know that 14 2 {\displaystyle {\frac {14}{2}}} {\displaystyle {\frac {14}{2}}} and 35 5 {\displaystyle {\frac {35}{5}}} {\frac {35}{5}} simplify to 7 {\displaystyle 7} 7. When you do the basic math for the other two ratios in the set, you find that both 10.5 1.5 {\displaystyle {\frac {10.5}{1.5}}} {\displaystyle {\frac {10.5}{1.5}}} and 24.5 3.5 {\displaystyle {\frac {24.5}{3.5}}} {\displaystyle {\frac {24.5}{3.5}}} also simplify to 7 {\displaystyle 7} 7, so that's your answer. Since all the ratios in the set have the same constant and the values are all increasing, you also know that this series is directly proportional.
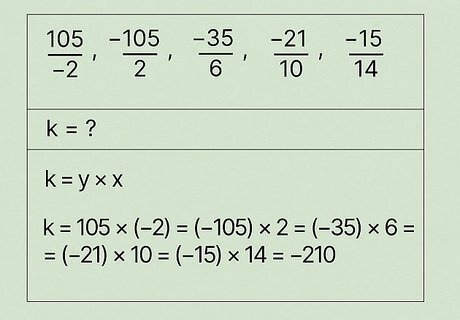
The constant of proportionality is − 210 {\displaystyle -210} {\displaystyle -210}. Since the y {\displaystyle y} y values are decreasing, you simply multiply the numbers together to get a value for the constant of proportionality, k {\displaystyle k} k using the equation k = y x {\displaystyle k=yx} {\displaystyle k=yx}. Before you even start multiplying, you can already tell that each of the ratios is going to simplify to a negative number. All that's left is to multiply each ratio to see that they all simplify to − 210 {\displaystyle -210} {\displaystyle -210}: For 105 − 2 {\displaystyle {\frac {105}{-2}}} {\displaystyle {\frac {105}{-2}}}, k = ( 105 ) ( − 2 ) = − 210 {\displaystyle k=(105)(-2)=-210} {\displaystyle k=(105)(-2)=-210}. For − 105 2 {\displaystyle {\frac {-105}{2}}} {\displaystyle {\frac {-105}{2}}}, k = ( − 105 ) ( 2 ) = − 210 {\displaystyle k=(-105)(2)=-210} {\displaystyle k=(-105)(2)=-210}. For − 35 6 {\displaystyle {\frac {-35}{6}}} {\displaystyle {\frac {-35}{6}}}, k = ( − 35 ) ( 6 ) = − 210 {\displaystyle k=(-35)(6)=-210} {\displaystyle k=(-35)(6)=-210}. For − 21 10 {\displaystyle {\frac {-21}{10}}} {\displaystyle {\frac {-21}{10}}}, k = ( − 21 ) ( 10 ) = − 210 {\displaystyle k=(-21)(10)=-210} {\displaystyle k=(-21)(10)=-210}. For − 15 14 {\displaystyle {\frac {-15}{14}}} {\displaystyle {\frac {-15}{14}}}, k = ( − 15 ) ( 14 ) = − 210 {\displaystyle k=(-15)(14)=-210} {\displaystyle k=(-15)(14)=-210}. Since all of the ratios have the same constant of proportionality, they are inversely proportional.

Flash will go 30 {\displaystyle 30} 30 feet in 5 {\displaystyle 5} 5 minutes and complete the 100-yard dash in 50 {\displaystyle 50} {\displaystyle 50} minutes. Start this problem by finding the constant of proportionality. You know you're going to be working with directly proportionate ratios because the more minutes pass, the further Flash will go, so you use the equation k = y x {\displaystyle k={\frac {y}{x}}} {\displaystyle k={\frac {y}{x}}}. The distance Flash goes depends on how long he's moving, so distance is the y {\displaystyle y} y variable and time is the x {\displaystyle x} x variable. You've been told that Flash goes 6 {\displaystyle 6} 6 feet in 1 {\displaystyle 1} 1 minute, so that makes his constant of proportionality 6 {\displaystyle 6} 6 ( k = 6 1 = 6 {\displaystyle k={\frac {6}{1}}=6} {\displaystyle k={\frac {6}{1}}=6}). To find how far Flash will go in 5 {\displaystyle 5} 5 minutes, use the equation for direct proportionality, y = k x {\displaystyle y=kx} y=kx. Here, x {\displaystyle x} x is 5 {\displaystyle 5} 5, so your answer is 30 {\displaystyle 30} 30 ( y = ( 6 ) ( 5 ) = 30 {\displaystyle y=(6)(5)=30} {\displaystyle y=(6)(5)=30}). To find out how long it will take Flash to complete the 100-yard (300 feet) dash, use the same equation, but solve for x {\displaystyle x} x instead. Start with 300 = 6 x {\displaystyle 300=6x} {\displaystyle 300=6x}, then divide each side by 6 {\displaystyle 6} 6 to get your answer, 50 {\displaystyle 50} {\displaystyle 50}.

Your car gets 28 {\displaystyle 28} {\displaystyle 28} miles to the gallon. The numbers for gallons of gas aren't in sequential order, but if you shuffle them around, you see that the values for both gas and miles driven are increasing—so this is a direct proportionality problem. The number of miles you drive depends on the gallons of gas you have in your car, so miles driven is the y {\displaystyle y} y variable, and gallons of gas is the x {\displaystyle x} x variable. Now, all you need to do is plug those values into the equation for direct proportionality: k = 224 8 = 28 {\displaystyle k={\frac {224}{8}}=28} {\displaystyle k={\frac {224}{8}}=28} k = 280 10 = 28 {\displaystyle k={\frac {280}{10}}=28} {\displaystyle k={\frac {280}{10}}=28} k = 112 4 = 28 {\displaystyle k={\frac {112}{4}}=28} {\displaystyle k={\frac {112}{4}}=28}
How can you tell if ratios are proportionate?
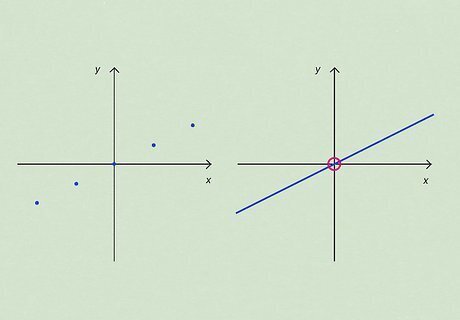
Plot the points on a graph and draw a line through the points. If the line you drew crosses the origin of the graph, the points have a proportional relationship. But if the line doesn't go through the origin, the points are not proportional. When graphing ratios, the first number (or numerator of a fraction) is the y {\displaystyle y} y coordinate and the second number (or denominator of a fraction) is the x {\displaystyle x} x coordinate.
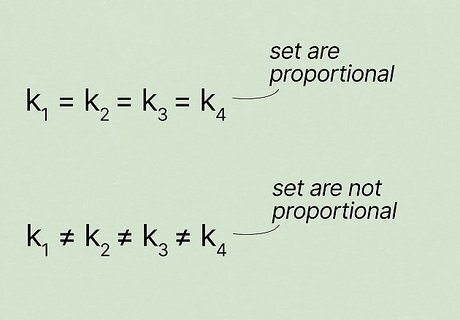
Find the constant of proportionality for each ratio. If any of the ratios in the set has a different constant of proportionality than the others, the ratios in the set are not proportional. But if all of the ratios have the same constant of proportionality, then they are proportional. Remember to use k = y x {\displaystyle k={\frac {y}{x}}} {\displaystyle k={\frac {y}{x}}} if the values of both variables go up and k = y x {\displaystyle k=yx} {\displaystyle k=yx} if the value of one variable goes up and the other goes down.























Comments
0 comment